Hillwoods academy, preet vihar SPACE club celebrated the winter solstice in a different way! This year we decided to do eratosthenes experiment and local noon experiment in tandem with another brach of our school in Gandhinagar, Gujrat where the school happens to be very close to tropic of Cancer! We planned to do the local noon finding at both the places of winter solstice day with gnomons of different shapes and sizes to make it more interesting. Mr. C B Devgun, President SPACE was invited on 20thDecember to give a talk on the winter solstice day and what students can do on that day. He interacted with students of SPACE club and other students and suggested them to find local noon with shadow formation of various objects.
On the day of winter solstice, around 6 teams from Hillwoods preet Vihar and 4 teams from Hillwoods Gandhinagar took the readings of the local noon. Hillwoods academy preet vihar team was under the guidance of Babita Kundalia, Coordinator, SPACE club. Various gnomons like gnomon stick provided by SPACE, basket ball pole, statue of liberty model, cricket stumps, road sighs were used to take the shadow measurements and students really enjoyed doing the shadow experiment! Hillwoods academy teams found the sunangle to be 52 degrees and Gandhinagar students found it to be 47 degrees as reported by them in evening. That turns out to be a difference of 5 degrees in latitude in both the schools (which was later verified from google earth)!! Local noon timings between both the schools were found to be around 18 minutes which converts to 4.5 degrees in longitude difference. Earth circumference was also measured taking these readings into consideration. A detailed report with readings will be soon made and sent.
We are attaching the images from the activity in the Hillwoods academy preet vihar. Pictures from the Gandhinagar branch are awaited. Students really enjoyed doing the experiment and will repeat the same again during equinox days and summer solstice day. We would like to thank SPACE for providing the technical support for the event.
STEPL (Space Technology and Education Pvt. Ltd.) conducted an experiment for school children as part of the Winter Solstice Day celebrations at Jantar Mantar on 22nd Dec by SPACE.
The competition titled 'Scientist of the Day' involved measuring the shadow of a long stick over a period of time and using the shortest shadow to find the Circumference of the Earth. This uses the concept that the sun rays shine directly overhead on the Tropic of Capricorn (the latitude line at 23.5° south, passing through Brazil, South Africa, and Australia) on the day of Winter Solstice. Younger students participated in a competition "Measuring the Sun Angle" which was a smaller part of the original one.
About 150 students from 21 schools from Delhi NCR participated in this competition, including schools such as The Airforce School, Subroto Park; DPS Sonepat; Ryan International School, Rohini; Step by Step, Noida; Apeejay School, Saket, etc. The students sat in groups amidst the green lawns surrounded by the ancient monuments and measured away for a couple of hours. It was a great experience for the teachers and students to be involved in such a project.
All the groups did a great job and attended to the measurements with attention and diligence. I am proud to announce the winners of these two competitions:
In Senior Section Ryan International School, Vasant Kunj won the first prize where as team 8 and team 7 from Ryan International School, Rohini got second and third prize respectively.
In Junior Section team 5 from The Air Force School, Subrato Park won the first prize where as team 15 and team 16 from Step By Step Greater Noida stood second and third prize respectively.
SPACE celebrated Winter Solstice with public outreach and with a large group of school students at Jantar Mantar, New Delhi22nd December was the shortest day of the winter, i.e. the Winter Solstice when the Earth's axis in the Northern Hemisphere was tilted furthest away from the sun. After this day, the day lengths slowly increase again. This day is celebrated over the world in different cultures, the most famous celebrations being at Stonehenge. SPACE observed this day in Jantar Mantar in New Delhi.
SPACE conducted public outreach at Jantar Mantar. Thankfully, although Winter Solstice indicates the peak of winter unofficially, it turned out to be a glorious sunny day. A lot of tourists and general wanderers walked through and were happy to find out what the ancient 'Jantar's (Yantras- meaning Machines) or structures were actually used for and how they were used to measure locations and time using the sun's shadow or by observing celestial objects.SPACE conducted tours of the various instruments and explained their workings.
STEPL (Space Technology and Education Pvt. Ltd.), i.e. the education wing of SPACE conducted an experiment for school children. The competition titled 'Scientist of the Day' involved measuring the shadow of a long stick over a period of time and using the shortest shadow to find the Circumference of the Earth. This uses the concept that the sun rays shine directly overhead on the Tropic of Capricorn (the latitude line at 23.5° south, passing through Brazil, South Africa, and Australia) on the day of Winter Solstice. About 200 students from 15 schools from Delhi NCR participated in this competition, including schools such as The Airforce School, Subroto Park; DPS Sonepat; Ryan International School, Rohini; Step by Step, Noida; Apeejay School, Saket, etc. The students sat in groups amidst the green lawns surrounded by the ancient monuments and measured away for a couple of hours. It was a great experience for the teachers and students to be involved in such a project.
Experimental material such as Telescopes, Binoculars and Astrokits were provided by SPACE Arcade www.spacearcade.in.
SPACE will celebrate Winter Solstice at Jantar Mantar
22nd Dec, 11:00am - 3:00 pm
SPACE will celebrate Winter Solstice with Public Outreach at Jantar Mantar, New Delhi.
Learn about all the Jantar Mantar instruments and see how they are used.
STEPL will conduct competitions on 'Measuring the Sun Angle' (Primary students) and 'Measuring the Circumference of the Earth' (Middle and Senior school students)
A solstice is an astronomical event that happens twice each year, when the tilt of the Earth's axis is most inclined toward or away from the Sun, causing the Sun's apparent position in the sky to reach its northernmost or southernmost extreme.
On the day of Winter Solstice, North Pole tilts away from the Sun and South Pole tilts towards the Sun. The Winter Solstice occurs exactly when the earth's axial tilt is farthest away from the sun at its maximum of 23° 26'. So the Sun shines at lowest heights in Northern skies and at maximum heights at Southern skies. It results in the shortest day in the Northern Hemisphere but at the same time it’s the longest day in Southern Hemisphere. So for people in Southern Hemisphere it’s a Summer Solstice.
Winter Solstice indicates winter at its peak. After this, the length of the day starts increasing and it reaches a point where day and night becomes equal in length at Vernal or Spring Equinox. The day continues to grow longer till Summer Solstice, the longest day.
The Winter Solstice will take place at 23:38 UT on 21st Dec (5:08 am IST on 22nd Dec) . In New Delhi, sunrise on winter solstice day is at 7:10 am and sunset is at 5:29 pm making it a day which is about 10 hours in duration.
On Dec 22nd, SPACE will conduct a Public Outreach in collaboration with Nehru Planetarium at Jantar Mantar, New Delhi. The students from various schools of Delhi will be performing activities tracking the shadow created by the sun using the Jai Prakash Yantra, and participating in competitions.
Since ancient times people were curious about the place they were living on ….The mother planet- Earth. After discovering the shape of earth, i.e. round, there were very few people who could think of measuring its size. Eratosthenes was one who first measured the circumference of the earth quite accurately.
Eratosthenes Background and his Experiment
Eratosthenes was a Greek living in Alexandria, Egypt, in the third century, BC. He knew that on a certain day at noon in Syene, a town a considerable distance to the south of Alaxandria, the sun shone straight down a deep well. This observation meant that the sun was then directly overhead in Syene, as shown in Figure 1. Eratosthenes also knew that when the sun was directly overhead in Syene, it was not directly overhead in Alexandria, as shown in Figure 2. Notice in both drawings, the sun’s rays are shown as parallel.
Figure 1 Figure 2
How Eratosthenes Found the Circumference of Earth
He was the first person to actually execute the idea of measuring circumference of earth and give the radius of the earth quantitatively. His idea was very simple.
He first made some simple assumptions:
·The earth is spherical
·The distance to the sun is very large compared to the size of the earth
·So the rays of the Sun falling on the earth are all parallel to each other
How did he do it, more than two thousand years ago? Take a look at Figure 3. Syene is represented by point “S,” and Alexandria by point “A”. In Figure 3, the arc length between S and A is d, and the angle corresponding to the arc SA is angle Q. The radius of Earth is R.
Eratosthenes perform the experiment at noon time. As he knew about the amazing fact about the zero shadow happening at Syene. It was very clear in his mind that the shadow can be zero only at the noon time. At other timings of the day we will certainly find some or the other shadow because of the daily movement of the sun. So, to find out whether the shadow becomes zero at Alexandria as well one had to observe it only at the noon time.
He observe at point S (in Syene) sun rayis perpendicular to the surface of Earth so casting no shadow and the sun is directly overhead there as shown in figure 4.
Therefore :
Noon shadow length at Syene on summer solstice day = 0 and sun is overhead.
He also measured the shadow of a tower A in Alexandria at noon shown in Figure 4.
The tower at A, which is perpendicular to Earth’s surface, and the ray of sunlight at point S both point to the center of Earth, and the rays of sunlight are parallel. So the angle between the sunlight and the tower is equal to Q (Alternate interior angles are equal.) Therefore: Q = Angle between Gnomon and Sun ray
Eratosthenes hired a person who walked from Alexandria till Syene and measured the distance, which cames out to be 718 km. Therefore :
d = Distance from the place where sun is overhead
If he had walked around the whole earth this curve would be 360 0 and the length he walked would be the circumference of the earth.
Therefore one can easily find out circumference of earth by using simple unitary method. According to this method if angle between Alexandria and Syene is 7º which is 50th part of the total angle at the centre of the earth…then the distance between the above two places is also just 50th of the total circumference. This can be written as the following formula:
Circumference of earth(C)/distance between two places(d)= 360º / angle between Gnomon and sunray(angle Q)
Rearranging for the circumference C, C = 360/Q X d
Measuring the Circumference of Earth by you at Jantar Mantar
You will also measure the circumference of the earth using the same method as Eratosthenes did. He measured it on the day of summer solstice but you will be measuring circumference of our planet on the day of equinox using the same technique.
So far you must have understood that, Eratosthenes used unitary method which is quite simple to implement. You only need to know the angle between place where you are doing the experiment and the place where sun is overhead and distance between these two places. Putting these values in the following formula will give us circumference of earth:
C = 360/Q X d
Let us now see how you will find he circumference of earth at Jantar Mantar -
Experiment
Material needed
Gnomon with base X 1
A plumb line (tie a nut with a thin thread) X 1
Drawing board X 1
White chart paper, sharp pencil, fine thread, board pins, protector, meter scale
Spirit Level X 1
India Map X 1(along with the scale mentioned on it)
PREPARATION:
Fix the chart paper on drawing board with the help of board pins
Unscrew the gnomon stick from the base and measure the full length of Gnomon stick several times. Record the Average length in millimetres. This is the height of the gnomon, H
Stick the gnomon base (yellow colored plastic piece) on the board using board pins in such a way that it should not move throughout the observations.
Mark with pencil, the centre of the hole where you are going to screw in the gnomon.
Screw the gnomon stick back in base.
Make a plumb line by attaching weight to the end of a string and try to make the gnomon stick as vertical as possible. For this you may stick some paper padding under base of the gnomon.
Position the board under the sun in such a way that the shadow of the tip of the gnomon is falling on the chart paper only and has plenty of space to move around without going off the chart paper.
Marking the shadow
1. Between 11:30am to 1pm mark the tip the shadow formed by the gnomon every 5 minutes.
2. Remove Gnomon from board.
3. Measure the shortest distance from the curve to the base of the pencil in millimetres several times. Record the average. This is the length L of the shadow
Measuring the Sun Angle ‘a’:
It is very simple to measure the sun angle.After conducting the experiment and measuring the length of shortest shadow you need to draw a right angle triangle with sides proportional to the Gnomon height H and shadow length L. For this draw a horizontal line CP=L; Draw a line AC perpendicular to it at one end, of length H. Join AP.
Measure the angle CAP i.e. ‘a’. This is the angle between your location and the place where sun is overhead on equator.
Measuring the Distance ‘d’
As you will be doing this experiment on the day of equinox, you already know that the sun is traveling above the equator, which means you need to find the distance between your place and equator along the longitude.
Finding this distance is also very simple as you just need an Indian map along with its scale (All maps carry a scale written on it which vary from map to map, e.g. 1cm = 150Km). You need to find the distance between Delhi and equator along with the same longitude and then multiply that to the map scale. This will give you the value of ‘d’
Alternatively you may also measure it before hand with the help of ‘Google Earth’ or any other map. One map will also be given as a reference.
* We will provide you the facility of both, google map and a large map showing India and equator on the competition site as well.
Measuring Circumference:
Using the following formula you will measure the circumference of the earth:
C/d = 360/a
C = 360/a X d
Where C – circumference, a- sun angle, d- distance between Delhi and place where sun is overhead.
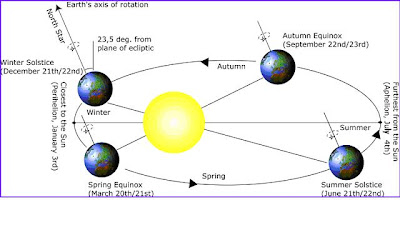
June 21 is a very important day for our planet in its relationship with the sun. June 21 marks the beginning of summer in the northern hemisphere and is called the summer solstice. It simultaneously heralds the beginning of winter in the southern hemisphere. It is the longest day for peopleliving in the northern hemisphere.In 2010, the solstice occurs in the Northern Hemisphere on June 21, at 11:28 UT, i.e. at 16:58 IST. The name is derived from Latin sol (sun) and sistere (to stand still), because at the solstice, the Sun appears to stands still in its movement during its motion (it appears to travel slowly).
The earth spins around its axis, an imaginary line going right through the planet between the north and south poles. The axis is tilted somewhat off the plane of the earth's revolution around the sun. The tilt of the axis is 23.5 degrees; and thanks to this tilt, we enjoy the four seasons. For several months of the year, one half of the earth receives more direct rays of the sun than the other half. Days are longer in the summer for the northern hemisphere due to the tilt of the Earth's axis allowing for more sunlight to be projected onto the surface.
At the June solstice, Earth is positioned in its orbit so that the North Pole is leaning 23-and-a-half degrees toward the sun. As seen from Earth, the sun is directly overhead at noon 23-and-a-half degrees north of the equator, at an imaginary line encircling the globe known as the Tropic of Cancer. The sun's rays are directly overhead along the Tropic of Cancer (the latitude line at 23.5° north, passing through Mexico, Saharan Africa, and India).This is as far north as the sun ever gets. This results in the longest day of the year. For example in New Delhi, sunrise on summer solstice day in 2010 is at 5:24 am and sunset is at 7:22 pm making it a day which is almost 14 hours duration.
When the axis tilts towards the sun, as it does between June and September, it is summer in the northern hemisphere but winter in the southern hemisphere. Alternatively, when the axis points away from the sun from December to March, the southern hemisphere enjoys the direct rays of the sun during their summer months. All locations north of the equator have day lengths greater than 12 hours at the June solstice. Meanwhile, all locations south of the equator have day lengths less than 12 hours.
June 21 is called the summer solstice in the Northern Hemisphere and simultaneously the winter solstice in the Southern Hemisphere. Around December 21 the solstices are reversed and winter begins in the Northern hemisphere.
Summer Solstice Event conducted by SPACE:
To celebrate summer solstice, SPACE will be performing public outreach at Jantar Mantar, New Delhi on Monday, 21st June. Educators from SPACE will be measuring the sun angle and the sun’s declination using the Ram Yantra and the Jai Prakash Yantra instruments. They will also explain the various instruments at Jantar Mantar to the public. All media and public are invited to attend this event.
Details:
Summer Solstice:June 21, at 11:28 UT, i.e. at 16:58 IST
Event: SPACE will conduct Public Outreach and Activities
Location: Jantar Mantar, New Delhi
Date and Time: Monday, 21st June, 2010 from 11:00 AM to 1:00 PM
For more information about the article/event contact Jaishree at SPACE at 9212669934.
This is the date (near March 20) when night and day are nearly the same length, and it marks the beginning of spring in the northern hemisphere. Equinox literally means "equal night". Come equinox day the sun shines directly on the equator and you have 12 hours of day and night, all around the world. However, the length of night and day across the world is nearly, but not entirely, equal - depending on location. As you observe, you come to realize that it is not the complete truth that every day the sun rises in the East and sets in the West. Equinox day offers opportunities to find facts about the way nature and science works in our lives.
Finding your location by just observing the sun movement in heavens is something unimaginable for a layman but we, astronomers can do that quite easily. March 20th is a BIG opportunity for us to find our location on the globe by observing the shadows formed by sun on that day. Working with hi-tech instrumentation and finding your coordinates is the thought which comes to your mind, but one can use sun and a small stick as his/her instruments and find the same. Of course you have to apply your brains to get meaning out the data you collect!!!! That shows us if you use logical reasoning and observe natural phenomenon carefully, you can find so many answers in science.
SPACE encourages students, teachers and school management to come forward and participate in this amazing experiment utilizing the phenomenon of Equinox. The activity and experiments would be conducted all across India in various centers of SPACE like schools, SNCs etc. The results from all the centres would be collated and each experiment team will be able to find the latitude of their location plus the difference in longitude corresponding to each other
Date: 20th March 2010
Timings: 11.00 am to 1 pm
Venue: School, home, public place
Experiments: Finding Local Noon and sun angle
So on 20th March observe the shadow formed by sunlight and find your location on Earth!!!
Equinox day 20th March 2010 Find your longitude and latitude by observing the shortest shadow
Name: School: SPACE club: Yes/No Space Nodal Centre: Yes/No Shortest shadow time (hr:min:sec): Sun Angle: School Address:
City: Telephone: e-mail: Other Details you want to put:
Send the completed Form fully filled back to SPACE office addressed to: SPACE, WZ-19, First Floor Asalatpur, A3 Block, JanakPuri, Delhi 110058 or mail the filled doc file to info@space-india.org
3. Drawing Board of Half imperial Size or 40cmx 40cm square board (husk, ply) 12 mm thick
4. A Sheet of white paper, pencil, fine thread, a nut or other suitable weight for plumb line
5. Spirit level
Preparation
1. Paste/ tack white paper on drawing board/square board.
2. Make the board horizontal by using the spirit level.
3. Measure length of Gnomon several times. Record the Average length in millimetres. This is the height of the gnomon, H.
4. Place the three legged base centrally on the board Fix with three pins.
5. Mark with pencil the centre of the hole in the middle
6. Assemble the gnomon rod into the central hole
7. Make a plumb line by attaching a the weight to the end of a string
Your gnomon is now ready for use
Marking the shadow
1. Find a site that is flat and open sky where the sun is visible between 11a.m and 1 pm (A terrace for instance SouthEast to SouthWest segment)
2. Find a flat surface. Place the base board and check with the spirit level to ensure that the surface is perfectly horizontal.
3. Place Gnomon with base on the surface. Adjust the base board so that the gnomon is vertical. Check using a plumb line. This is very important
4. Between 11 am and 1 pm mark the tip the shadow formed by the gnomon every 5 minutes. Ensure that the stick is vertical throughout the observations.
5. Remove Gnomon from board.
6. Draw a smooth curve joining all the points through all the observations
7. Measure the shortest distance from the curve to the base of the pencil in millimeters several times. Record the average. This is the length L of the shadow
8. Construct a similar right angle triangle with sides proportional to the Gnomon height H and shadow length L, using a large scale factor. For example draw a horizontal line AM=2L; Draw a line
AP perpendicular to it at one end, of length 2H. Join PM.
9. Measure the angle MAP. This is the angle between the vertical in your location and the direction of the noon day sun. This is the Sun angle and on the day of the equinox, this is the latitude of your location. The angle MPA is the altitude of the sun at the time of local noon.
See attached images for further clarification. For detailed information see demonstration video on the activity at youtube.
When we want to know the time, we consult a watch. The time the watch gives us is called the Indian Standard Time. The life of our country is regulated by this time. We all know that this time we use in our day to day life is the local time of a place which is on a longitude which divides India into two halves in the east-west direction. That place is at 82.5 degrees E away from the Greenwich which is the zero longitude. 'E' shows that it is east of Greenwich. It falls in between two big cities, Allahabad and Varanasi. So whenever someone asks you the time, you look at you watch and tell him/her the time of the place at 82.5 degrees and not the time at your place. That's why when it is noon or rather the LOCAL noon at your place your watch does not show 12 o'clock in the afternoon! If you are east of the 82.5 degrees then your local noon will happen before the IST noon and vice versa for the person west of 82.5 degrees.
For finding more about the latitude and longitude, please refer to the accompanying doc “Latitude and Longitude”
This difference can be used to get your longitude. Lets see it this way. The whole earth is 360 degrees and it takes 24 hours by your watch to rotate back to same point after one rotation.
So 24 hours = 360 degrees
1 hour = 360/24 = 15 degrees
4 minutes = 1 degree
Now if two places are separated by 15 degrees in longitude on the earth, they will have a difference of 1 hour in their time. You already know that at the local noon the shadow of your gnomon is the shortest. So by measuring the difference (in time) in the local noon at two difference places, we can get the longitude difference.
In our experiment on 2oth March this year, we are going to measure the time of shortest shadow at our place and we have the time at which the shortest shadow forms at the meridian at IST (82.50E). Let this be T.
The time difference expressed in minutes and divided by 4 should give us gives us the difference it degrees in Longitude.
But there is a complication! The standard time refers to the local noon at 82.50 for a FICTITIOUS SUN! The earth DOES NOT go around the sun at a uniform rate! It moves faster in winter and slower in summer. Again the axis of rotation of the earth is tilted its orbit around the sun. Both these make the sun act like a clock that runs sometimes slow and sometimes fast! This is very inconvenient to say the least. So our day to day watch pretends that there is a fictitious sun that keeps uniform time throughout the year. The time it takes for the earth around the sun once is divided by 365 and then by 24 to define a standard hour. Standard times of all countries use this method.
So the local noon at 82.50 east occurs LATER than 12 o'clock on the watch on some days and EARLIER on other days! This correction, which changes throughout the year, is called the equation of time. Fortunately it is the SAME at ALL longitudes and latitudes and is known for every date. So it can be eliminated while taking the difference between IST meridian and our local meridian.
How to do it?
Get the local noon time in IST by looking at the watch (the time at which we get the shortest shadow in our place) “t “ by observing the shortest shadow formation by observing the gnomon shadow.
We know the local noon time at 82.50 as this is the IST we all use.
Local noon time on 20th March, 2010 at 82.50 =12:08 PM (this is calculated with ephemeris and softwares)
You can also do the same for any place on earth by using the following page on the internet:
Find (T-t) in minutes and divide by 4 to get the longitude difference.
Add or subtract ( if you are east of the IST timeline then add and if you are west to IST timeline then subtract from 82.50 E and find YOUR longitude!
In understanding how science works it is always much much better to assume concepts and the minimum number of known quantities. Then the measurement gives support to the CONCEPT assumed rather than claiming accuracy of a number which experts have already measured in any case! More than that it teaches us that cooperation is as IMPORTANT in science as it is in other aspects of life, if not more so.
If we place a gnomon at different locations on earth and closely watch the shadow during daytime, we will observe that the angle between the vertical and the mid day sun will be different at different places (or latitudes). Can we use this to find the latitude of a place? Or can we use this method to find out the difference in latitude for two different places? Should we walk all around the earth and find the circumference and then the radius of the earth to do so? Well we don’t have to go to so much trouble. Should we use a map with a scale that was made over hundreds of years by other people walking long distances and surveying the land? No! There is no need to do it. Should we copy the radius of the earth from a handbook and use it? No! No!
This is getting a bit irritating! Lets go to the heart of the matter right away. As before A and B are two locations on the surface of the earth of radius R. Two sticks (Gnomon) of equal length (AP=BQ) are placed in the two locations. The shadow length AM is shorter than shadow length BN. The direction of the mid -day sun at A and B are MP and NQ. The shorter shadow implies that the sun has a higher altitude at A than at B. The angle APM between the vertical and the direction of the sun at A can be measured by constructing similar triangles on paper or by using trigonometry. The angle BQN between the vertical and the direction of the sun at B, can be similarly measured
Look at the figure below. Let the circle below represent the earth. The vertical at A and B is defined as the line joining the centre of the earth to the points A and B respectively. The verticals through them are OAP and OBQ respectively. The shortest shadow is cast by the mid day sun. The rays of the sun falling on the earth may be considered parallel, as the sun is very far compared to the size of the earth. From measurement we know the angles APM and BQN and the difference between them. It is clear from the geometry that this is equal to the angle AOB subtended by the arc AB at the centre of the earth.
Suppose the direction of the noon day sun at station A is as shown in the diagram below
ie the sun is exactly overhead at noon at A and casts no shadow! The angle POQ is still the difference in the angle between the vertical angle of the noon sun at the two places. From simple geometry angle POQ = angle BQN, the vertical angle of the noon sun at B. So you can easily measure it at B. If station A is at the equator, it directly measures the LATITUDE at B! Now March 20 is the equinox day, On this the sun shines directly over the equator. If we perform the experiment by measuring the vertical angle of the noon day sun on that day everywhere in the world we can measure our own latitude!
WHAT are we assuming here?
The Earth is spherical.
The Sun is very very far as compared to the size of the earth
The sun shines exactly overhead on the equator on March 20, 2010, the equinox day. This we took from an Astronomy Handbook as the day when sun’s declination is 0 degrees.
We can also measure latitude on any day of the year. But that is another measurement and another adventure in science!
Any location on Earth is described by two numbers--its latitude and its longitude. If a pilot or a ship's captain wants to specify position on a map, these are the "coordinates" they would use. Actually, these are two angles, measured in degrees, "minutes of arc" and "seconds of arc." These are denoted by the symbols (0, ', ") e.g. 350 43' 9" means an angle of 35 degrees, 43 minutes and 9 seconds (do not confuse this with the notation (', ") for feet and inches!). A degree contains 60 minutes of arc and a minute contains 60 seconds of arc--and you may omit the words "of arc" where the context makes it absolutely clear that these are not units of time.
Calculations often represent angles by small letters of the Greek alphabet, and that way latitude will be represented by λ (lambda, Greek L), and longitude by φ (phi, Greek F). Here is how they are defined.
Latitude The latitude angle lambda Imagine the Earth was a transparent sphere (actually the shape is slightly oval; because of the Earth's rotation, its equator bulges out a little). Through the transparent Earth (drawing) we can see its equatorial plane, and its middle the point is O, the center of the Earth. To specify the latitude of some point P on the surface, draw the radius OP to that point. Then the elevation angle of that point above the equator is its latitude λ--northern latitude if north of the equator, southern (or negative) latitude if south of it.
[How can one define the angle between a line and a plane, you may well ask? After all, angles are usually measured between two lines! Good question. We must use the angle which completes it to 90 degrees, the one between the given line and one perpendicular to the plane. Here that would be the angle (900-λ) between OP and the Earth's axis, known as the co-latitude of P.]
Lines of latitude
On a globe of the Earth, lines of latitude are circles of different size. The longest is the equator, whose latitude is zero, while at the poles--at latitudes 900 north and 900 south (or -900) the circles shrink to a point.
Longitude On the globe, lines of constant longitude ("meridians") extend from pole to pole, like the segment boundaries on a peeled orange.
Every meridian must cross the equator. Since the equator is a circle, we can divide it--like any circle--into 360 degrees, and the longitude φ of a point is then the marked value of that division where its meridian meets the equator.
Longitude lines or "meridians"
What that value is depends of course on where we begin to count--on where zero longitude is. For historical reasons, the meridian passing the old Royal Astronomical Observatory in Greenwich, England, is the one chosen as zero longitude. Located at the eastern edge of London, the British capital, the observatory is now a public museum and a brass band stretching across its yard marks the "prime meridian." Tourists often get photographed as they straddle it--one foot in the eastern hemisphere of the Earth, the other in the western hemisphere. In the medieval times Ujjain was designated as zero longitude for Indian calculations of astronomical tables. Kanchipuram and Kurukshetra also served similarly
A lines of longitude is also called a meridian, derived from the Latin, from meri, a variation of "medius" which denotes "middle", and diem, meaning "day." The word once meant "noon", and times of the day before noon were known as "ante meridian", while times after it were "post meridian." Today's abbreviations a.m. and p.m. come from these terms, and the Sun at noon was said to be "passing meridian". All points on the same line of longitude experienced noon (and any other hour) at the same time and were therefore said to be on the same "meridian line", which became "meridian" for short.
